Ann Gardner’s hanging steel and glass sculpture, Ripple, was installed in Troxel Hall in 2013. It represents connections made during a person’s time in college and throughout life. The intertwining steel bars form the foundation and the attached square glass mosaic tiles reflect the diversity in life’s connections.

Viewed from the bottom, Ripple resembles the circle of life.  Viewed from the side it illustrates how connections may continue forever. Constructed with both contemporary (steel) and ancient (glass tiles) materials, Ripple visually embellishes the concept that connections have formed for centuries and will continue into the future.

Ann Gardner (b.1947 Eugene, Oregon) is a Northwest artist who creates glittering mosaics about repetition, pattern, and rhythm. She builds installations with a combination of concrete/metal and glass mosaics which she cuts and lays individually to develop complex patterns and abstract forms.

Gardner has explored and experimented with ceramic glassblowing. She imprints glass with texture of clay and produces ceramic vessels encrusted with patterned surfaces of kitchenware shards. These experiments led her to create installations with freestanding structures and wall-mounted and hanging sculptures embedded with pieces of glass. By employing natural light and incorporating existing architectural structures, Gardner’s sculptural mosaics, like Ripple, transform their surroundings and call attention to the passage of time by highlighting changing light conditions.

Gardner has received numerous commissions and is represented in many collections, including the Smithsonian American Art Museum in Washington, DC.  Gardner’s sculpture Ring of Water was recognized in 2004 as one of the best public art projects, receiving the Urban Design Award from Americans for the Arts. In 2008, Fog received the Juror’s Choice Award from the Corning Museum of Glass published in New Glass Review 29. In 2011, Gardener became the first glass mosaic artist to receive the Rakow Commission for Five Pods. In 1993 Gardner received a Louis Comfort Tiffany Award for talent and individualistic strength.

Below are the named installations and additional examples of Garner’s art.

Fog, glass, composite, concrete, steel

Relevant Terminology/History

Roman Tile Mosaics were typically made from small tiles that ranged between 0.5 and 1.5 centimeters in the shape of a square. Fine details were made using even smaller pieces (tesserae). These tiles were made using ceramics, pottery, marble, glass, glass paste, shells, and stone. Romans would create the design on a surface coated with new mortar. After the pieces were in place, the entire mosaic would be grouted and then polished and cleaned.

A Roman mosaic on a wall in the House of Neptune and Amphitrite, Herculaneum, Italy, 1st century AD – photo courtesy of Wikipedia 

Dr. Klemm visited El Jem, Tunisia during June 2023.  She photographed the Roman mosaic (approximate date of 250 CE) of the nine Greek muses and their description displayed in the El Jem Museum.  See her standing in the center of the El Jem Roman Coliseum, a UNESCO site. El Jem was known as Thysdrus at the time of the Roman Empire.

Glass was used in mosaics as early as the 3rd century BCE by artisans in Greece, Persia and handshakes.India.  Whereas clay tiles are dated as early as 8000 BCE, there were significant barriers to the development of glass tiles, including the high temperatures required to melt glass, and the complexities of mastering glass annealing techniques.

Networking is the process of making connections and building relationships. A person’s connections are often illustrated with spokes and dots.  The illustration below is a Venn-diagram like illustration of members on a team.

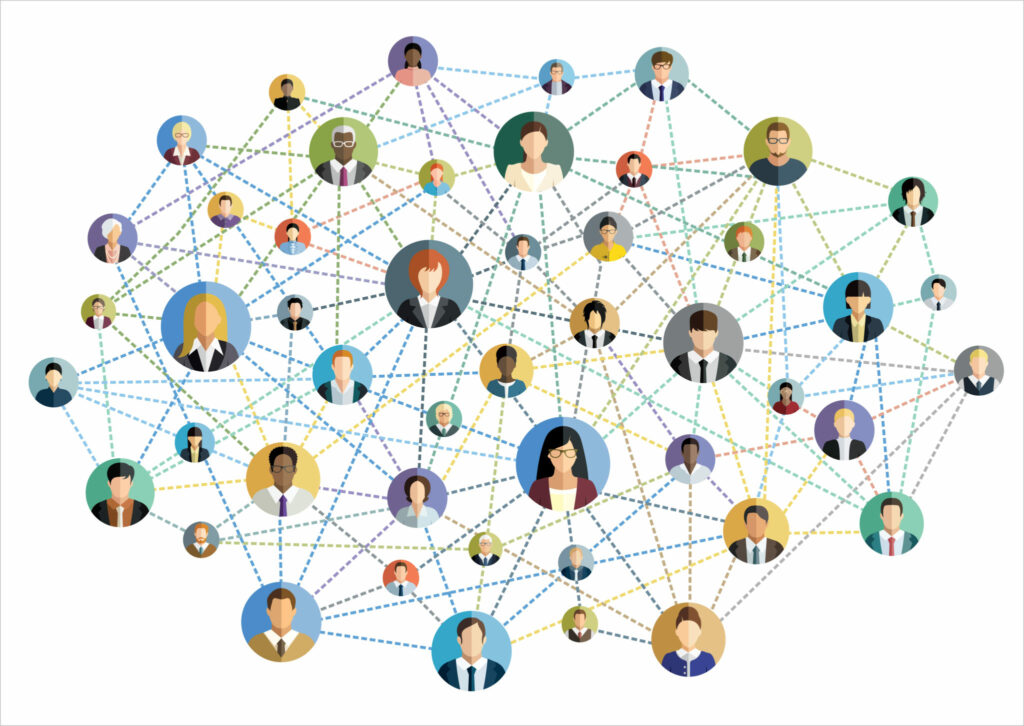
Image courtesy of CADM

Six degrees of separation is the idea that all people are six or fewer social connections away from each other.  The chain of “friend of a friend” statements can be made to connect any two people in a maximum of six steps or handshakes.  In the illustration below, Ryan is six degrees of separation from Arnold.

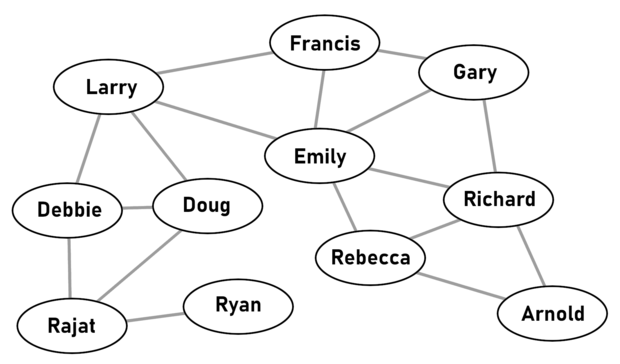
Image courtesy of Wikipedia

1. What do you think the title has to do with the art?
2. Why do you think Number Character 8 and STEM Squad member ∞ speak about the art?
3. How does the red image with white numbers relate to the art? 
4. How does the hidden blue image relate to the art?  What does it suggest about the viewer’s network?  How many degrees of separation is the viewer (“you”) to the other people?
5. How do the shapes of 8 and ∞ relate?
6. What are the usual shapes of Roman tiles?  What is the shape of the tiles in Ripple?
7. What was Louis Comfort Tiffany famous for?  Have you ever seen any of his art?
8. Who is in your network?  Why are you connected?  Will the connection last a long time? Why is this the last question?

NumbersAlive! is excited to announce that the linked organization, NumbersAlive! Foundation has been approved as an educational 501(c)(3) nonprofit organization. All donations received via the “donate” button or through formal communications can be deducted under IRS Section 170.

The Foundation will create experiences for families and community organizations and provide unique learning tools to students and schools with limited resources. Contact Dr. Klemm, if you wish to tailor your donation of $100 or greater to a specific program. Naming options are available for funding an episode(s) of the Global Traveling Numbers Show that allows students to participate in the development and production, day-or weeklong sessions of Building NumberOpolis!, professional training for parents and teachers, etc. If you wish to support making NumbersAlive!’s unique learning tools available to deserving students, parents or teachers, please contact Dr. Klemm: rebecca@numbersalive.org. One hundred percent (100%) of ALL donations will go to programs or donations. Dr. Klemm will cover all administrative costs.