
About the Art
e+l+e+m+e+n+t+a+l was installed in 2010 on the exterior and lobby of Hach Hall, home of Iowa State University’s Department of Chemistry. Multiple panels of cobalt-blue backlit glass are mounted on aluminum panels on the west door and lobby incorporating chemical patterns and elements. The installation also includes Penrose tiling patterns that occur on microscopic surfaces, a reminder of chemistry’s connection to everything in the world. Incorporating Penrose patterns serves as a tribute to Iowa State chemists and materials scientists, including Dan Shechtman, PhD, an Iowa State Professor, and winner of the 2011 Nobel Prize in Chemistry for his discovery of quasicrystals.
The lobby includes a long glass case that is reminiscent of a traditional classroom building display case. Within the case are whimsical and evocative blown-glass that form chemistry-inspired sculptures, inviting playful inquisition. Some of the glassware are the familiar apparatus of a chemistry lab: pipette; funnels; and beakers. Others are instruments like the glass trumpet suspended from the top of the case. The dangling figure with sword is a glass version of a G-Nome “guardian” installed on the roof of the nearby Molecular Biology Building (see G-Nomes).
This sculptural display includes both pure elements and oxides. The title of the work, e+l+e+m+e+n+t+a+l, refers to the use of elements and molecular themes that are basic to chemistry. The word “elemental” also conveys something fundamental, deep, and basic, suggesting the essential role chemistry plays in our lives.


About the Artist
Norie Sato is a Seattle-based artist. Born in Japan in 1949, she immigrated to the United States as a young child. She received a Bachelor of Fine Art (BFA) in Printmaking in 1971 from the University of Michigan and a Master of Fine Art (MFA) in Printmaking and Video in 1974 from the University of Washington.
Sato creates both studio art and installations for public places. She works from site and context-driven ideas first, then finds the appropriate form and materials, striving to add meaning and human touch to the built environment. Sato installations can be explored in universities, airports, libraries, transit stations, city halls, convention centers, and parks all over the United States. See some of her art below or The Fifth Muse in this book.

Meet Me at the Triangles, 2020, Marion Fryer Town Square, Wheaton, MD

Ghost Palms, 2007, Miami International Airport, South Terminal International Baggage Claim, Miami FL

In the Fold, 2020, Viamonte Senior Living, Walnut Creek, CA
Relevant Terminology/History
Dan Shechtman is a Professor of Materials Science at Iowa State University who received the 2011 Nobel Prize in Chemistry for discovering quasicrystals.
Quasicrystals are stable phases of condensed matter that exhibit crystalline features such as symmetry and repeating patterns of unit cells.

Image of quasicrystals courtesy of Wikipedia.
Tiling or tessellation is the covering of a surface using one or more geometric shapes, called tiles, with no overlaps and no gaps.
Penrose tiling is a non-periodic tessellation filling a plane without translational symmetry – the pattern cannot be shifted to match itself over the entire plane. Any bounded region, no matter how large, is repeated an infinite number of times within the tessellation.

Image of a Penrose courtesy of Wikipedia.
*Fun Fact: In 2023 a new 13-sided shape was uncovered as the first example of an elusive “einstein” — a single shape that can be tiled infinitely without repeating a pattern.
David Smith, a retired printing technician from East Yorkshire, England came across the solution for the einstein.” He went to academic mathematicians to verify what he discovered. How did he come across this remarkable solution? “I’m always messing about and experimenting with shapes,” Smith told The New York Times. “It’s always nice to get hands-on. It can be quite meditative.”
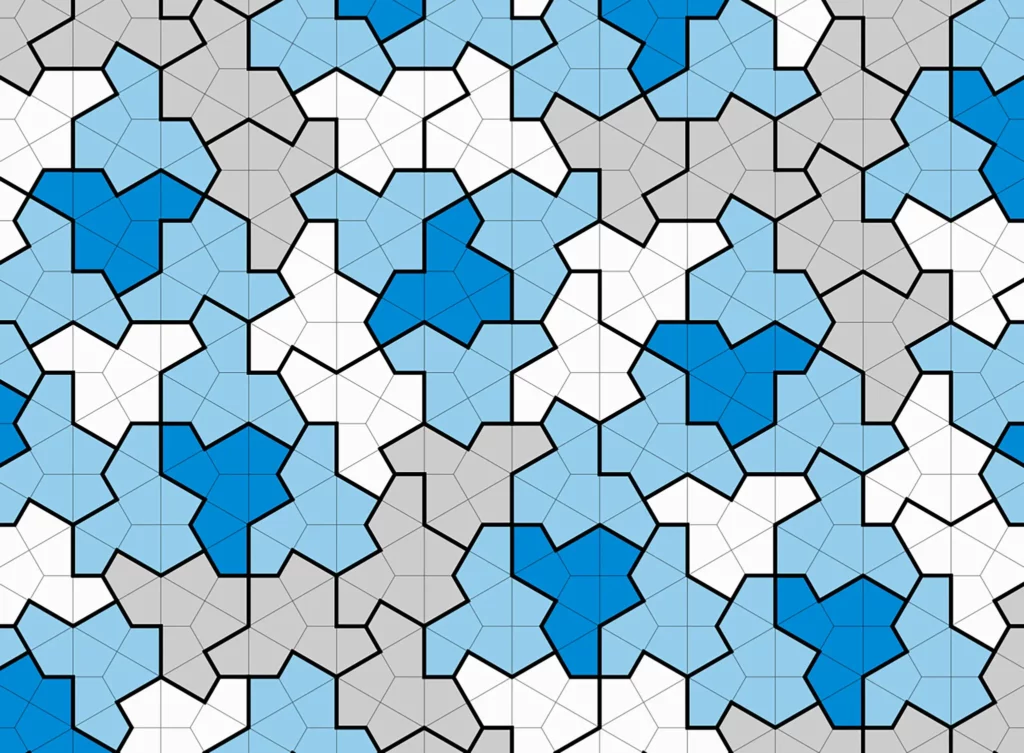
Image courtesy of the New York Times.
Periodic table of the elements is an arrangement of the chemical elements into rows (“periods”) and columns (“groups”). It is an organizing icon of chemistry and is widely used in physics and other sciences. It is a depiction of the periodic law — when the elements are arranged in order of their atomic numbers an approximate recurrence of their properties is evident. The table is divided into four roughly rectangular areas called blocks. Elements in the same group tend to show similar chemical characteristics.
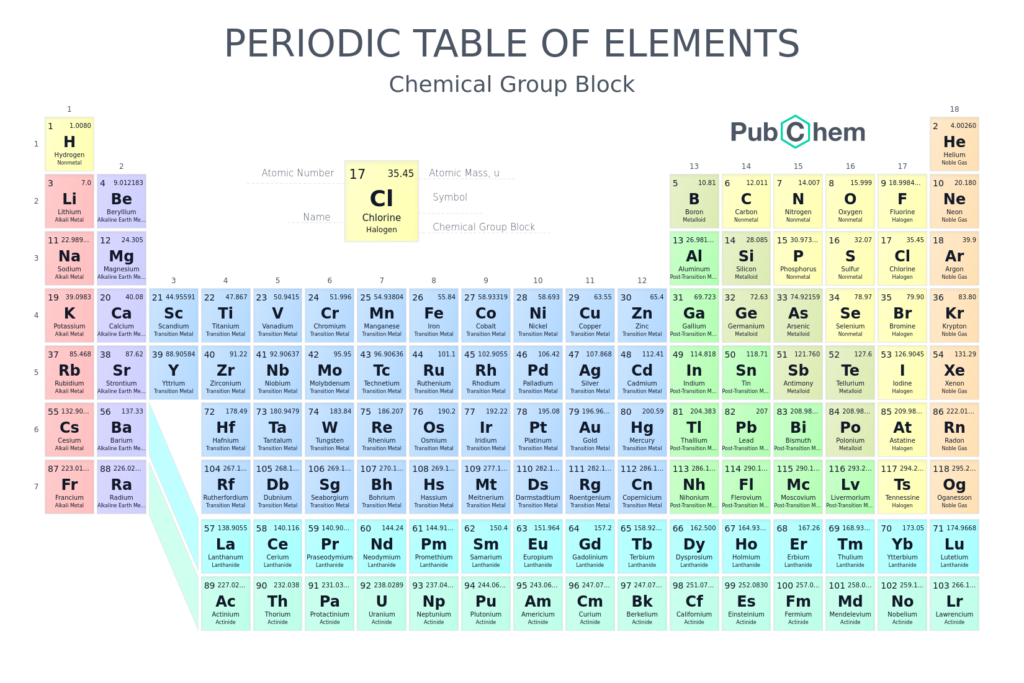
For more information, visit: https://en.wikipedia.org/wiki/Periodic_table.
Learning Guide
- What do you think the title has to do with the art?
- Why do you think Number Character 2 is speaking about the art?
- How do the red images with white numbers relate to the art? Can you find the shapes in the art?
- How do the hidden blue images relate to the art?
- How did you respond to the question posed by Number Character 2? How did you make your decision?
- What folk tale is Number Character 2 referring to with the mirror?
- What patterns do you notice in the design of the quasicrystal in the image above?
- What patterns do you notice in the design of the Penrose image above? What shapes create the tiling/tessellation?
- When you took Chemistry in school, was the Periodic Table of the Elements the same as the one provided above? If not the same, what difference(s) do you note and what created the change(s)?
- Do you think there are any missing elements? Why or why not?
- Look at the “einstein” tiling presented in March 2023. Let it serve as a reminder that mathematics is a living subject with ideas that anyone can discover if you continue to play with math and explore ideas using your hands and mind. Printing technician, David Smith, called it the “the hat.” What would you call it instead?
- What is the interior pattern of each 13-sided hat?
- If a space is the shape of the 13-sided polygon hat, would you call the interior pattern a tiling?
